Here is a great numeracy task from Peter Liljedahl’s website.
Adam, Bob, Clair and Dave are out walking: They come to rickety old wooden bridge. The bridge is weak and only able to carry the weight of two of them at a time. Because they are in a rush and the light is fading they must cross in the minimum time possible and must carry a torch (flashlight,) on each crossing.
They only have one torch and it can’t be thrown. Because of their different fitness levels and some minor injuries they can all cross at different speeds. Adam can cross in 1 minute, Bob in 2 minutes, Clair in 5 minutes and Dave in 10 minutes.
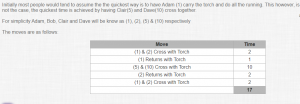
Adam, the brains of the group thinks for a moment and declares that the crossing can be completed in 17 minutes. There is no trick. How is this done? [from Nigel Coldwell]
Here is a solution to this task (found on Nigel Coldwell’s site):